Research

Current Projects

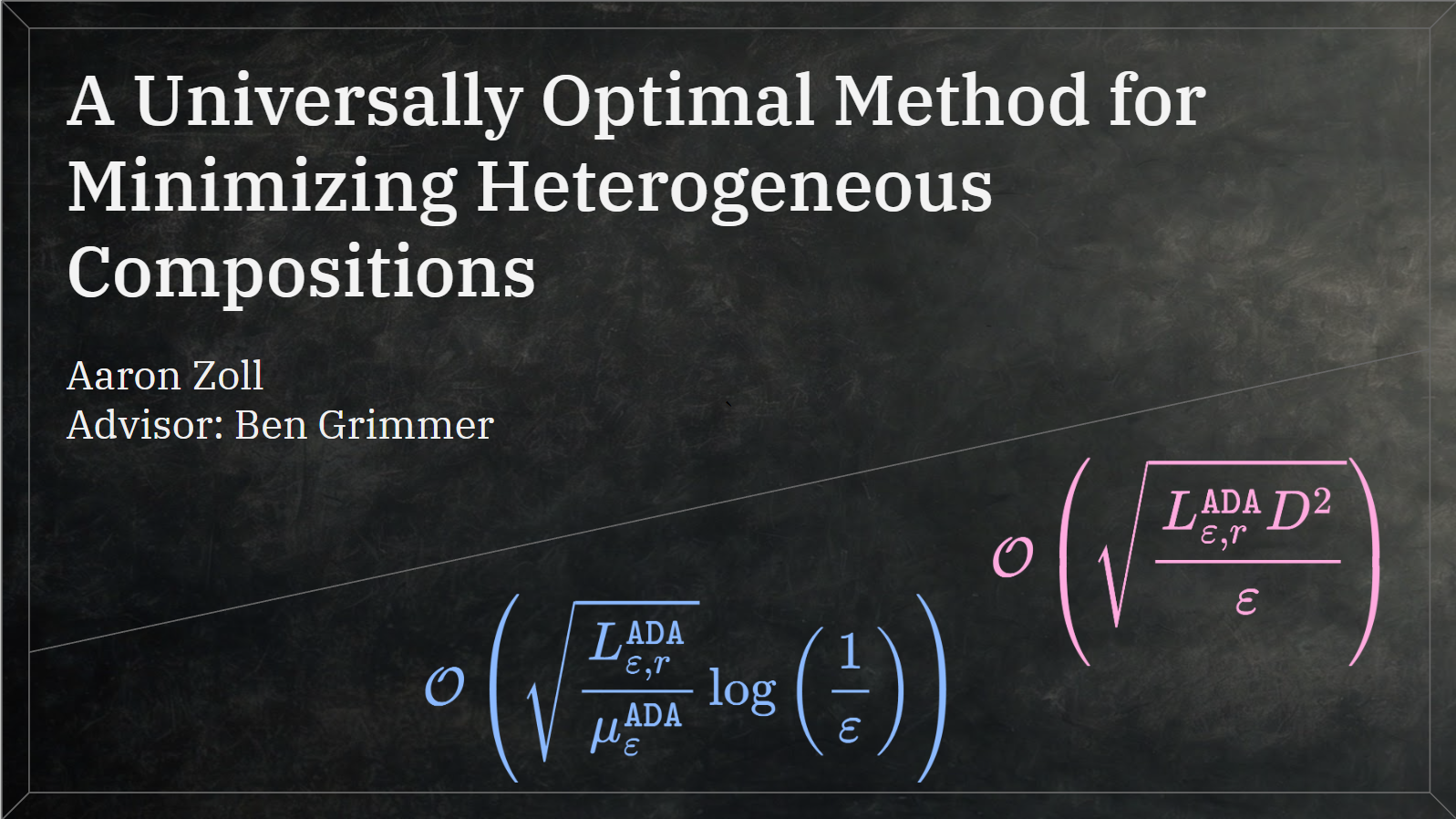
My current research aims to construct and design universally optipal methods for minimizing a wide class of functions, bridging the gap between smooth and nonsmooth optimization. Previous work has dealt with compositions of Hölder smooth functions, with interpolate the smoothness between Lipschitz functions and those with Lipschitz gradient (typically denoted as “\(L\)-smooth”). Current work aims to further analyze the complexity of first-order algorithms on functions that may only attain an approximate notion of smoothness.

Papers (preprints)

• A Universally Optimal Primal-Dual Method for Minimizing Heterogeneous Compositions arXiv

Slides

• Presentations on Heterogenous Compositions

  • Johns Hopkins Applied Math and Statistics Student Seminar
  • Jr MINDS seminar, an event hosted by The Johns Hopkins Mathematical Institute for Data Science (MINDS) encouraging grad students to share their research.
  • International Conference on Continuous Optimization (ICCOPT)